Quantum Bootcamp Part II: A Tough Nut to Crack

In this second installment of “Quantum Bootcamp,” our new column on acquiring a grounding in quantum computing (here’s the first column), economics concentrator Ethan Wang ’26 describes his deepening commitment to the complexities of quantum mechanics. Under the tutelage of the Department of Chemistry’s Benjamin Lienhard, a postdoc in the Rabitz Lab, Wang and ECE major Wonju Lee ’26 ponder the Lagrangian, the Hamiltonian, and the Principle of Least Action on the long and challenging approach to a succinct description of classical mechanics.

We began the process of acquiring a background in quantum computing a few months ago, meeting in Ben’s office on the second floor of Frick Lab. Our weekly sessions consisted of reviewing the chapter we had read over the previous week, going over additional exercises, and engaging in discussions about general quantum or physics concepts that were not covered in the book.

I quickly realized that our first text, Leonard Susskind’s The Theoretical Minimum (Basic Books, 2014), is more suited towards reviewing concepts already taught in prior education rather than building a working knowledge of the subject from scratch. This was especially evident in the mathematical explanations and derivations of certain concepts. Even though I took multivariable calculus just last semester—and the material is still fresh in my mind—I saw that it would be difficult to follow.

The beginning of Susskind’s book was accessible enough, especially as it was related to classical high school physics concepts we had already learned and were plenty familiar with. One of the first challenges we encountered was when Ben asked us to take a crack at the derivation of the equation for a harmonic oscillator. I discovered that the process involved a painstaking amount of algebraic guesswork, i.e., picking sin or cos to model a key behavior of that oscillation based only on the knowledge that they model oscillatory behavior in certain ways, or start at certain amplitudes.

Past Chapter 6, “Principle of Least Action,” the explanations got very technical and mathematically oriented, even as the written sections gave rough outlines and general key takeaways. It really helps to have a strong math background for this kind of work. It would have made things easier if I also had more theoretical physics in my background to begin with.

At the same time, this chapter introduced a series of related concepts that are cross-applicable to quantum mechanics and thus are particularly useful and relevant for our purposes.

To begin with, a fundamental building block is the idea of a Lagrangian, which is a function of the position and velocity of a system that, when integrated over an interval, provides the action. By setting the action to stationary according to the principle, we arrive at the Euler-Lagrange equations and then the Hamiltonian.

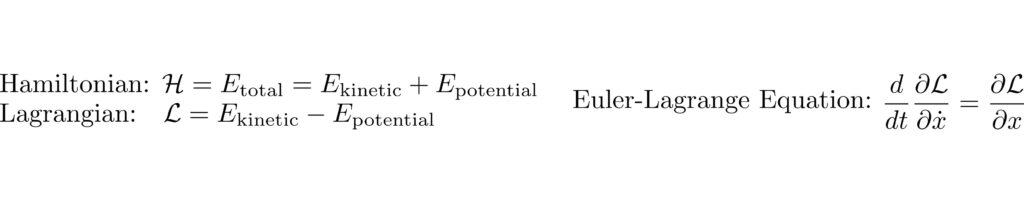
The Hamiltonian represents the total energy of a system—the sum of the kinetic, E_kinetic, and potential energy, E_potential—while the Lagrangian equals the difference of the kinetic and potential energy. Along with the Lagrangian and the Principle of Least Action, it helps form a way of describing systems that is both minimalistic (in the sense that the same simple principles always apply) and consistent (in that different reference frames can be factored in to still yield correct results).

Basically, it makes describing a system itself simple. I found this to be interesting and somewhat ironic—while you achieve elegance on the broader level, there is a ton of complexity still hidden inside each variable or small symbol that encompasses all the caveats you bought this simplicity with.

Ultimately this sort of simplicity is most useful for the purposes of physicists, but it often makes decoding the dense equations a more arduous ordeal.

The profound quality of these equations will help us when we are actually investigating quantum systems and need to describe their behavior in a concise way. But actually fitting physical behavior to these equations—as we learned from our harmonic oscillator tangent and as Ben tells us—is often a guessing game of trying various trial functions to see what ultimately best approximates observations and the corresponding mathematical description.

Another important concept was the idea of phase spaces and reversibility. In essence, the Hamiltonian way of doing physics allows us to find exact trajectories if we are given exact coordinates and momenta, tracing the different states that evolve over time from an initial state. The preservation of the volume of phase space is a somewhat abstract way to represent different states where ultimately you can plot these states in a graph based on the coordinates of position and momentum and have them be “incompressible.” This incompressibility is known as Liouville’s theorem, and while we can just take it to be gospel for now without totally justifying it to ourselves, eventually it will parallel something known as unitarity. Unitarity is very important to quantum operators.

An interesting fact we came across that even Ben found novel was that Poisson Brackets have no real deeper meaning as a physics concept apart from being shorthand for a specific equation expressing the effect of the order of derivation of two functions.

Having finished the book, we are still nowhere close to any sort of mastery of classical mechanics—which is to be expected. Even the book itself admits to being a “minimum.” However, we are now aware of much more of the framework that physics uses to describe the world in as elegant a way as possible.

As we delved into quantum mechanics, we saw many parallels that would otherwise have been lost on us and felt as though we were working on a pre-existing base instead of finding ourselves in the deep end right away.

Next time, we will cover a discussion Wonju and I had after just finishing Susskind’s next book, Quantum Mechanics—The Theoretical Minimum (Basic Books, 2015).

Some sources for enrichment: 

Online textbook section about Hamiltonian from LibreTexts/Chemistry

A digestible video about Lagrangian and Hamiltonian from YouTube’s “Physics with Elliot”

Principle of Least Action from YouTube’s “Physics with Elliot”